Printable version

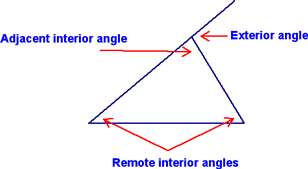
Proving that Lines are Parallel - Lesson 5-2

Today, we covered a number of theorems regarding how to prove that lines are parallel. In order to prove these, we started by explaining what an exterior angle was:

You should be able to recognize that there are 6 exterior angles for every triangle. We then covered a number of theorems that can be proved based on the first (#30). The proof for Theorem 30 and the others, which follow directly from that, are in the book...be sure that they make sense to you!!

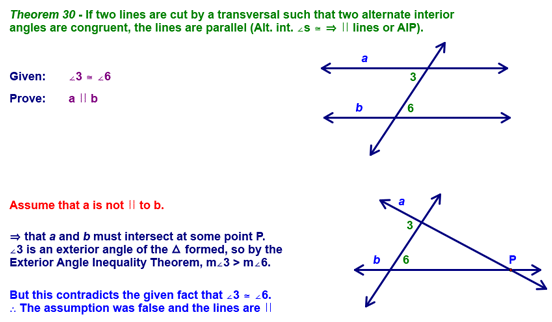
We did prove one of these in class...Theorem 31. The others are very similar.

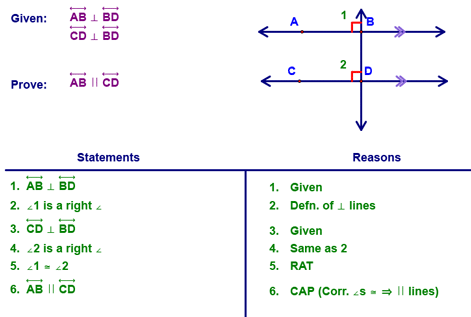
You should be able to see how you could prove AEP & CAP by a similar method.

At this point, we covered a number of other theorems:

And wrapped up by doing an example (which is the proof for Theorem 35):